|
Phi From The Sky. Crop Formation Spirals. Part 1. By Jack Sullivan Some of the most spectacular crop formations have been spirals or have used partial spirals in their structural design. Some serious investigators have remarked that they see a relationship to the Golden Number ‘Phi’ but this suggested relationship rarely seems to be adequately explained. This article is an attempt to remedy this situation as well as identifying other types of spirals found in crop formations in past years. Below are twenty four images seen at various times over the last twenty years or so. You can find others in the archives of various crop formation web sites. My thanks to all the individual photographers who have provided these shots over the years and who of course hold the copy write.
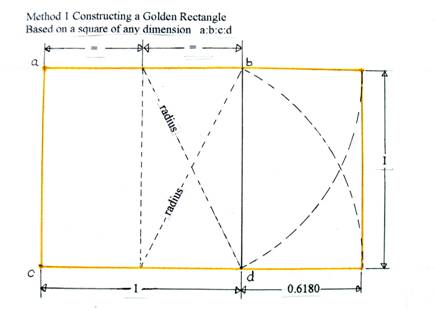
So what is Phi? It is a numerical ratio and its mathematical value is equal to the square root of five plus one, divided by two. ( See Construction Method 2 for geometrical proof of this statement.). The square root of five is an irrational number, i.e. it does not have a terminating decimal. Therefore Phi also is an irrational number. For practical purposes the value used is 1.6180. For more information, Google Search “Phi The Golden Number” In this article this numerical value is not required suffice to say that it is known as the Golden Ratio which then gives rise to The Golden Rectangle and The Golden Spiral as explained below. The Golden Rectangle and The Golden Spiral The Golden Rectangle is an oblong which has sides of lengths in the proportion of Phi, i.e. they are in the ratio of 1.6180 to 1. We require only a set square or a ruler and a pair of compasses to create the oblong. No actual measurements are required, only a little practical geometry. There are two known simple methods Method 1. Create a square. Draw a straight line of any chosen length, and use the compasses set to this length to strike off arcs from each end that are parallel to the line. Draw a further line tangential to the first one and using the set square erect a vertical end line joining the two horizontals. Use the compasses as already set to mark a point along one of the horizontals and draw the second vertical to complete an accurate square. See illustration below. Using the compasses bisect one of the horizontals and draw a line dividing the square into two exactly equal parts then draw a diagonal joining the corners of one of these parts., see illustration. Set the compasses to the exact length of the diagonal and from an inner corner of the half square, describe an arc beyond the outer edge of the square. Repeat this operation but now placing the point of the compass at the opposite inner corner of the half square to strike a second arc in line with the first one and connect the two arcs with a tangential line and extend two sides of the original square to produce the golden rectangle which will have sides proportional to the Phi ratio. This can be verified by measurement of the dimensions on the drawing.
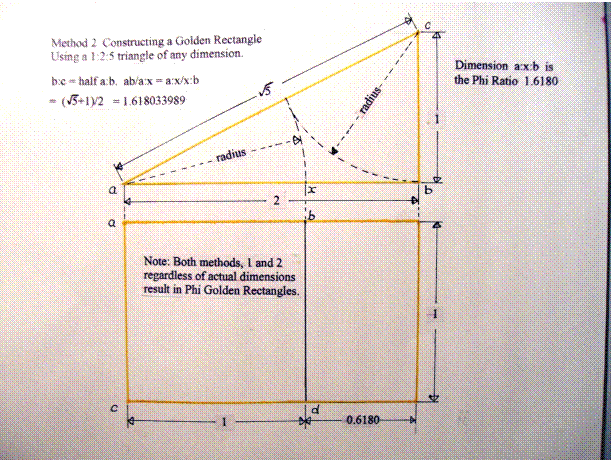
Generating the Golden Rectangle Method 2. The Golden Triangle. Draw a horizontal line of any convenient length and bisect it. Set the compasses to exactly one half of the line length and use this measure to make the vertical line b:c. Draw the hypotenuse of the triangle 1:2: 5 back down to point ‘a’. With the compass still set at length b: c: describe the arc from point b to meet the hypotenuse and with the compass reset from this point to point a describe the arc to give point x, see illustration below. The original horizontal line is now divided into lengths a:x: and x:a . These are proportionate to the Phi ratio and enable the construction of the Golden Rectangle projected below it. A Pythagoras Theorem states that in any right angled triangle, The Square on the Hypotenuse is equal to the sum of the squares on the other two sides. In the illustration the hypotenuse, side a.c. is the square root of 5. This is derived from the addition of side a.b. 2 squared = 4, to side b.c squared = 1, giving the total of five. Taking the square roots of each of the sides gives the dimensions shown in the illustration. The ratio of a.b. 2 to the other two sides is 2 : ( 2.33606 =1) which is equivalent to 1 : 3.33606
divided by 2. This gives us the Golden Phi ratio of 1 :
1.6180.
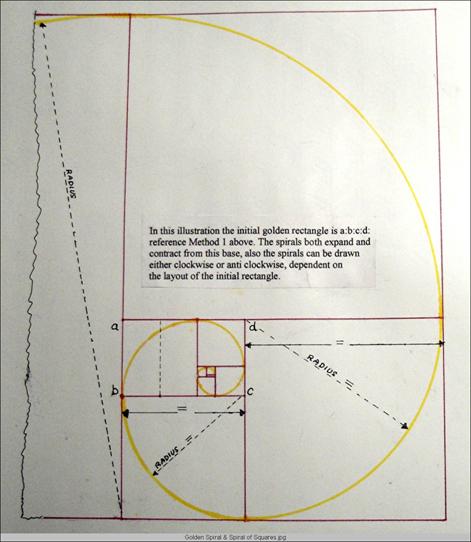
Referring back to Method One we can see that the Method Two Triangle a; b: c: with its hypotenuse, equivalent to the square root of five (2.2360) is contained four times in the initial square a; b: c: d: confirming the truth of Method Two. Using the Golden Rectangle to develop a spiral of squares for a true Golden Spiral. The Phi rectangle has the unique property of being self replicating. It is divided into a true square with always a remaining portion which is a golden rectangle in itself, see illustration below. From any golden rectangle a progression can be made to either lesser or greater dimensions which will in the first instance, create a spiral of squares, enabling circular quadrant arcs to be struck in each of the squares which combine to form a smooth Phi Golden Spiral curve. Note that each of the squares (red outlines) has the Phi relationship to both a preceding one or a successive one. Larger squares are 1.6180 times bigger than the preceding square and smaller squares are 0.6180 times smaller than the preceding square. Illustration of Phi Spiral Generation using a Spiral of Squares or ‘Tiles’.
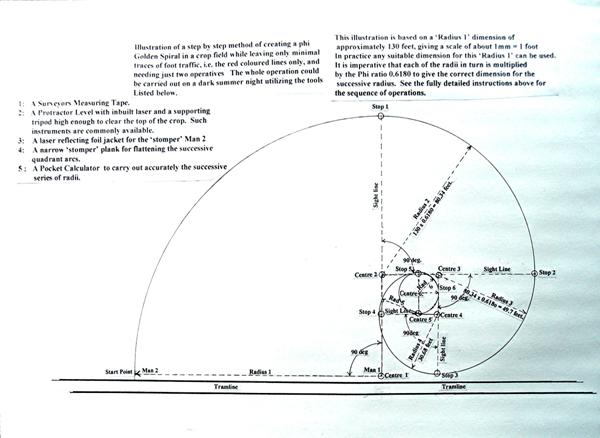
This mathematical relationship enables the use of calculation rather than geometry to generate a Golden Spiral. A straight line of any length considered as a radius in the above diagram, can be the basis of the radius for either the preceding quadrant arc radius or a successive quadrant arc radius. Measure your straight line and for an increasing quadrant arc radius multiply by 1.618. For a decreasing quadrant arc radius multiply by 0.6180. This procedure greatly simplifies the creation of any Phi Golden Spiral of any required size in any media, including say a field of crop. As an exercise, the following is a detailed explanation with an accompanying illustration of how such a crop formation could be created in real time with minimal disturbance to the standing crop. The necessary tools for the job are listed within the drawing. Sequence of operations for creating a Phi Golden Spiral of any convenient size in a typical English wheat crop field in darkness, using 2 men only, and tools as listed. In the illustration. The drawing below shows that whatever dimension is selected for Radius 1.the total dimension across the spiral will be radius 1 + radius 2. This dimension is also the product of radius 1 multiplied by 1.6180, the Phi ratio. If radius 1 is 100 foot the maximum width of the finished spiral will be 161.8 feet, 1: A straight section of tram line is chosen and Man No.1 holding the reel of surveyors tape and with laser level set upon the tripod, positions himself two or three feet to the side of this at centre 1. 2: Man No. 2, carrying his narrow stomper plank, takes the end of the tape and walks down the tram line to a distance of 100 feet measured as accurately as possible, He to then steps outside the tramline to the start point. The tensioned tape is now parallel to the tram line and this mans reflective foil jacket will be visible to Man No. 1 caught in the beam of the aligned laser. 3: Man No 1 now swings the laser beam through 90 degrees, aiming at the position of the first Stop Point. and Man 2 stomps the first quadrant arc to this position, where he will find himself illuminated by the laser beam, and stays there until further instructed by Man No.1 4: Man No.1, guided by the laser beam , now moves directly towards Man No. 2 treading carefully through the standing crop to minimize damage and winding in tape until the distance between him and Man No 2 reduces to 61.8 feet at Centre 2. He then plants his tripod and swings the laser through again 90 degrees aiming at Stop 2. and instructs Man No. 2 to carry on stomping the second quadrant arc using the tensioned tape as the radius, until caught in the reset laser beam 5: Man No.1 Now repeats the procedure from Operation 3 onwards, moving to each new Centre Point in turn.. Man 2 stomps the quadrant arcs successively but stopping when illuminated by the reset laser beam until Man 1 reels in the surveyors tape to the next required radius dimension. The radius dimensions will have been pre-calculated by simply multiplying each successive radius by the Phi ratio 0.6180 and listed by Man 1 to be checked off as the Centre points are reached during the progress of the work. The foot traffic trace created by Man No.1 will form a decreasing spiral of straight lines (marked in red on the drawing) and the procedure can be terminated at any Stop Point by using the next radius dimension to create a complete circle. Illustration of a Potential Method of Field Generation of a Phi. Spiral, with minimum disruption to the standing crop. Would be hoaxers take note.
The Stone Henge Julia Set of July 1996 ( see below), the spine of which is a near perfect Phi Golden Spiral, is believed to have appeared between the hours of 4pm to 6pm in an afternoon. ( Reports available via a Google Search) The illustration above could be used if your ambition runs to creating a replica of this spectacular crop formation, be aware that the creation of the graduated discs and the attached pairs of ‘legs’ present a enormous further complication in that all the disc’s diameters will need to be calculated exactly to produce the perfection of the outlining spiral curvatures. The Stone Henge Julia Set of July 1966.
Phi From The Sky. End of Part 1. |